Trees¶
Definition
A tree is a collection of nodes. The collection can be empty; otherwise, a tree consists of
- a distinguished node \(r\), called the root.
- zero or more nonempty subtrees \(T_1, \dots, T_k\), each of whose roots are connected by a directed edge from \(r\).
Definition
- Degree of a node: number of subtrees of the node.
- Degree of a tree: maximum among the degrees of all nodes.
- Parent: a node that has subtrees.
- Children: the roots of the subtrees of parent.
- Siblings: children of the same parent.
- Leaf (terminal node): a node with degree \(0\) (no children).
- Path from \(n_1\) to \(n_k\): a unique sequence of nodes \(n_1,n_2,\dots,n_k\) such that \(n_i\) is the parent of \(n_{i+1}\).
- Length of path: number of edges on the path.
- Ancestor of a node: all the nodes along the path from the node up to the root.
- Descendants of a node: all the nodes in its subtrees.
- Depth of \(n_k\): length of the unique path from the root to \(n_i\).
- Height of \(n_k\): length of the longest path from \(n_i\) to a leaf.
- Height / Depth of a tree: the height of the root or the maximum depth among all leaves.
Representation¶
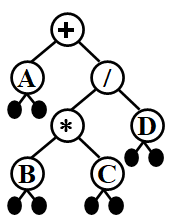
Consider the following tree.
List Representation¶
- Array Representation
- Linked List Representation
FirstChild-NextSibling Representation¶
This representation is not unique with different FirstChild chosen.
Binary Tree¶
Definition
A binary tree is a tree in which no node can have more than two children.
Since it has the same structure of FirstChild-NextSibling Representation, thus all trees can be represented by binary trees.
Property
- In a binary tree, left child and right child are different.
- The maximum number of nodes of level \(i\) is \(2^{i - 1}\). The maximum number of nodes in a binary tree of depth \(k\) is \(2^k - 1\).
- \(n_0 = n_2 + 1\), where \(n_0\) is the number of leaf nodes and \(n_2\) is the number of nodes of degreee 2.
typedef int ElementType;
typedef struct TreeNode {
ElementType element;
struct TreeNode *left;
struct TreeNode *right;
} Tree;
Traversals¶
There are four traversals for a binary tree, preorder traversal, inorder traversal, postorder traversal and levelorder traversal (or in graph, breadth first search, BFS).
- Pre-, in-, and postorder traversals are implemented by recursion, or alternatively by a stack with iteration.
- Levelorder traversal is implemented by a queue.
Tip
For a general tree, it also has
- Preorder Traversal: first itself, then sons.
- Postorder Traversal: first sons, then itself.
- Levelorder Traversal.
void Preorder(Tree *tree) {
if (tree == NULL) {
return;
}
printf("%d ", tree->element);
Preorder(tree->left);
Preorder(tree->right);
}
void Inorder(Tree *tree) {
if (tree == NULL) {
return;
}
Inorder(tree->left);
printf("%d ", tree->element);
Inorder(tree->right);
}
void Postorder(Tree *tree) {
if (tree == NULL) {
return;
}
Postorder(tree->left);
Postorder(tree->right);
printf("%d ", tree->element);
}
void Levelorder(Tree *tree) {
Enqueue(queue, tree);
while (!IsEmptyQueue(queue)) {
Tree *cur = Dequeue(queue);
printf("%d ", cur->element);
Enqueue(queue, cur->left);
Enqueue(queue, cur->right);
}
}
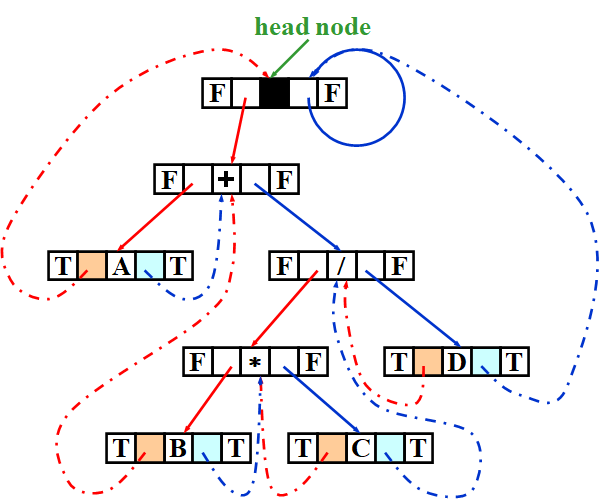
Threaded Binary Trees¶
There are \(n + 1\) NULL children of the leaf nodes of a binarty tree with \(n\) nodes. We want to make these NULL pointers more useful. And here comes the Threaded Binary Trees.
typedef int ElementType;
typedef struct ThreadedTreeNode {
ElementType element;
struct ThreadedTreeNode *left;
struct ThreadedTreeNode *right;
bool leftThread; // `true` to indicate `left` is a thread instead of a child pointer.
bool rightThread; // `true` to indicate `right` is a thread instead of a child pointer.
} ThreadedTree;
Rule for Threaded Binary Trees
NOTE: the following "inorder"" can also be replaced by any traversals.
Rule 1: If tree->left == NULL, then replace it with a pointer to its inorder predecessor.
Rule 2: If tree->right == NULL, then replace it with a pointer to its inorder sucessor.
Rule 3: A threaded binary tree must have a head node of which the left child points to the first node.
Binary Search Tree (BST)¶
Definition
A binary search tree is a binary tree. It may be empty. If it is not empty, it satisfies the following properties:
- Every node has a key which is an integer, and the keys are distinct.
- The keys in a nonempty left subtree must be smaller than the key in the root of the subtree.
- The keys in a nonempty right subtree must be larger than the key in the root of the subtree.
- The left and right subtrees are also binary search trees.
ADT
Objects: A finite ordered list with zero or more elements.
Operations:
- Make an empty BST.
- Find a key in a BST.
- Find the minimum key in a BST.
- Find the maximum key in a BST.
- Insert a key to a BST.
- Delete a key in a BST.
BST
Tree *Find(Tree *tree, const ElementType element) {
if (tree == NULL) {
return NULL;
}
if (element == tree->element) {
return tree;
}
return Find(tree, elemet < tree->element ? tree->left : tree->right);
}
Tree *FindMin(Tree *tree) {
if (tree == NULL) {
return NULL;
}
return tree->left == NULL ? tree : FindMin(tree->left);
}
Tree *FindMax(Tree *tree) {
if (tree == NULL) {
return NULL;
}
return tree->right == NULL ? tree : FindMax(tree->right);
}
Tree *Insert(Tree *tree, const ElementType element) {
if (tree == NULL) {
tree = (TreeNode *)malloc(sizeof(TreeNode));
tree->element = element;
tree->left = tree->right = NULL;
} else {
if (element < tree->element) {
tree->left = Insert(tree->left, element);
} else {
tree->right = Insert(tree->right, element);
}
}
return tree;
}
Tree *Delete(Tree *tree, const ElementType element) {
if (tree == NULL) {
// Warning: Element not found.
return NULL;
}
if (element == tree->element) {
if (tree->left != NULL && tree->right != NULL) {
TreeNode *temp = FindMin(tree->right); // (1)!
tree->element = temp->element;
tree->right = Delete(tree->right, temp->element);
} else {
TreeNode *temp = tree;
tree = tree->left != NULL ? tree->left : tree->right;
free(temp);
}
}
if (element < tree->element) {
tree->left = Delete(tree->left, element);
} else {
tree->right = Delete(tree->right, element);
}
}
- Here it's replaced with the smallest node in the right subtree. Or alternatively it can be replaced with the largest node in the left subtree.
Time Complexity¶
The average complexities of all operations are \(O(d)\), where \(d\) is the depth of the target node. The average depth over all nodes in a tree is \(O(\log N)\).
But for the worst cases, when building a tree by inserting a set of oredered elements, it will degenerate to linear list with \(O(N)\) complexity of each operation.
AVL Tree¶
Remains
Splay Tree¶
Remains
B-Tree¶
Remains
创建日期: 2022.11.10 01:04:43 CST